Ashley knots; ABOK #2217 covering knot

For those of you who aren’t familiar with blue collar knots, “ABOK” means “The Ashley Book of Knots,” an ecyclopedic book on knots written in 1944 that has since become the reference for knot identification. I think it was my maternal grandfather that gave me my, somewhat rare, now the worse from love and use, 1st edition copy back in the 1960s; but what with the natural self-naturedness of a boy I cannot recall for sure. #2217 refers to a particularly handsome knot in the chapter “The Monkey’s Fist and Other Knot Coverings.” To justify “handsome” requires a sample photo from cbrew6 on Knot Heads World Wide:

This knot is “tied on the table,” which means that one uses a diagram to draw it. From a topological point of view, a table diagram in this case is a mapping of the surface of the sphere to the plane. A line drawing shows the path that the cord takes. The path is a loop, that is, it ends at the same point at which it starts. The path is restricted to never cross itself twice at a single point. At each crossing, some sort of notation indicates which line is to be on top, but for planar knots like the above, it is arranged so that the cord will weave over, under, over, under … And it is a trivial fact of practical folks topology that one can always assign such a pattern.

The above knot is “5-ply,” by which is meant that the knot was tied once and then duplicated four times by following the initial path over again four times. This is made convenient by the fact that the end point for the knot is the same as the initial point. The difficulty in designing knots like these is to arrange this, that is, one wishes the path to be a single loop.

ABOK #2217 has a 4-fold symmetry axis. In the above example, the axis runs vertically, roughly between the two purple cords extending through the top. This allows us to show how it was designed, perhaps, and to redraw its tying diagram into a form that is easier to generalize. The 4-fold axis is around the center of the tie diagram:

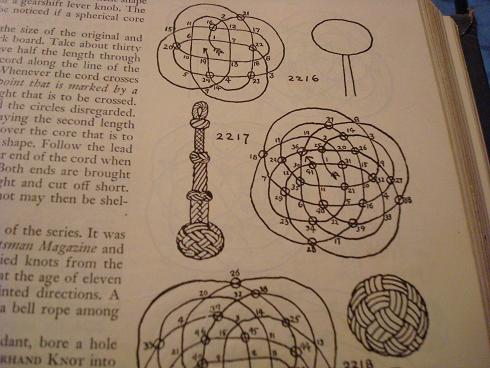
In the above knot, the bights are made of 3 or 4 cords. This is ideal for a covering knot; a larger number would tend to let the encased object peak through:

A convenient way of designing a knot is to assume that it has some symmetry and to draw a region that can be stitched together several times. In stitching together two regions, we will simply number the free ends from each region and connect them in order.

Since the knot is going to have at least one 4-bight, we might as well assume that the knot has a 4-fold axis through a 4-bight. The regions we’ll stick together will need to be approximately diamond shaped, with a height around half their width:

The diamond shaped regions need to be approximately flat. This means that they should be constructed from 4-bights, that is, squares. And this construction works for knot #2217. The diamond shaped pattern is:

The diamond shaped region has 7 ends on the left and 7 ends on the right. Numbering these in order, we find that the diamond pattern defines a permutation which is a 7-cycle, (1475263):

Furthermore, the mapping takes rising lines to rising lines except for the top and bottom two rows. This ensures that there aren’t any sudden direction changes in the middle regions.

To get the 2217 knot, we use the above diagram four times. In fact, a single cycle knot would result if we reproduced it N times providing N is not a multiple of 7. More generally, for this sort of scheme to work, we need for the section of the knot to be an M-cycle if it has 2M free ends, and we need to reproduce it a number of times that is relatively prime to M. This is the equivalent of a well known result for the Turk’s heads. Ashley managed to write out a table classifying Turk’s head knots by number of bights and number of leads for up to 40 leads and 24 bights (in our example, there are 4 bights and 7 leads) but didn’t quite manage to figure out the relatively prime detail. As close as he could come was “A good practical way to plan Turk’s Heads is to take a prime number for the larger dimension (5, 7, 11, 13, 17, 19, 23, 29, 37, 41, etc.) and to use any smaller number, either odd or even, for the other dimension.” This quote is from ABOK # 1314. An example of several Turk’s heads, along with an ABOK #2217, see: Bell rope with ABOK #2217. For those who aren’t knotees, I should mention that white cord is the most difficult to tie as it shows errors most easily.

So, drawn in Turk’s head form, here’s my simplified diagram for the ABOK #2217:

This means we now also have a decent covering knot with a 3-fold axis of symmetry instead of 4. Such a knot has 30 crossings where #1314 has 40. Does the ABOK list such a knot? No, we may have found a new covering knot, assuming that it’s not already in the literature. But in any case we had fun and the method should generalize to produce other beautiful knots.

P.S. For any sort of plane (or spherical surface) polygonal region, Euler characteristic tells us that V-E+F = 2 where V = the number of vertices, E = number of edges, and F = the number of faces. For planar knots, E = 2F so this reduces to V = F+2. For this knot, V=10B, F = 10B+2, where “B” is the number of bights. For ABOK #2217, B=4.

For an arbitrary convex polygon with all vertices having four faces meet (as applies to a loop) and assembled from 4A triangles and B squares, V = 3A+B, E= 6A + 2B, F = 4A+B so we have (3A+B)-(6A+2B)+(4A+B) = 2 or A=2. So there will be 8 triangles and B squares. This suggests that the most symmetric covering knot will be one that distributes the 8 triangles as evenly as possible around the sphere. For knot ABOK #2217, the 8 triangles are distributed with a 4A2 symmetry. A more symmetric symmetry would be cubic, with the triangles on the corners of the cube. That is approximately obtained when the #2217 is tied with three bights instead of four. Of the 8 triangles, two are on the north and south poles. The rest alternate equally spaced above and below the equator. This is as close as you can get to cubic symmetry:

I may tie a 3-bight knot and put a picture up here. And sorry for the rough diagrams, WordPress has just changed their software and it defaults to medium resolution on my efficient little PNG files.

P.S. The “Turk’s Head” method of tying ABOK #2217 and variations works quite nicely. I’ll put up a series of photos showing how to do this soon. And the 3-bight version is a pretty knot: